When studying the diffusivity of non-electrolyte solutes in liquid solutions, especially at a very dilute level, two main correlations are typically used: the Wilke-Chang correlation, the Hayduk-Laudie correlation. Also, Taylor-Duss suggest an expansion to the mentioned correlations.
Wilke-Chang Correlation
This method helps to estimate the binary diffusivity of a solute (A) in a solvent (B). It’s represented by Equation (24-52) in the Fundamentals of Momentum, Heat, and Mass Transfer textbook by Welty et al.. To use this equation, you need the following:
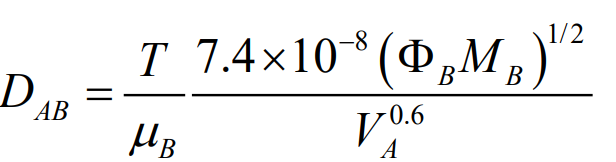
- DAB: Binary diffusivity of A in B (in cm^2/s).
- T: Absolute temperature in Kelvin.
- µB: Viscosity of solvent B in centipoise.
- MB: Molecular weight of solvent B.
- ΦB: Association parameter of solvent B.
- VA: Molal volume of solute A at its normal boiling point.
To find the necessary molecular weights and volumes, refer to Tables 24.4 and 24.5 in the mentioned textbook. If data for computing the molar volume of solute at its normal boiling point, VA, are not available, Tyn and Calus recommend the correlation:
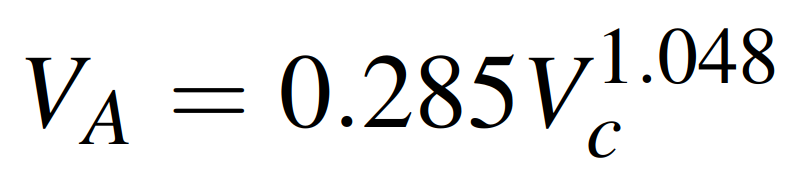
where Vc is the critical volume of species A in cm3/g. mol. Values of Vc are tabulated in the properties of gases and liquids book, Appendix A.
For the association parameter of common solvents, see the table below.
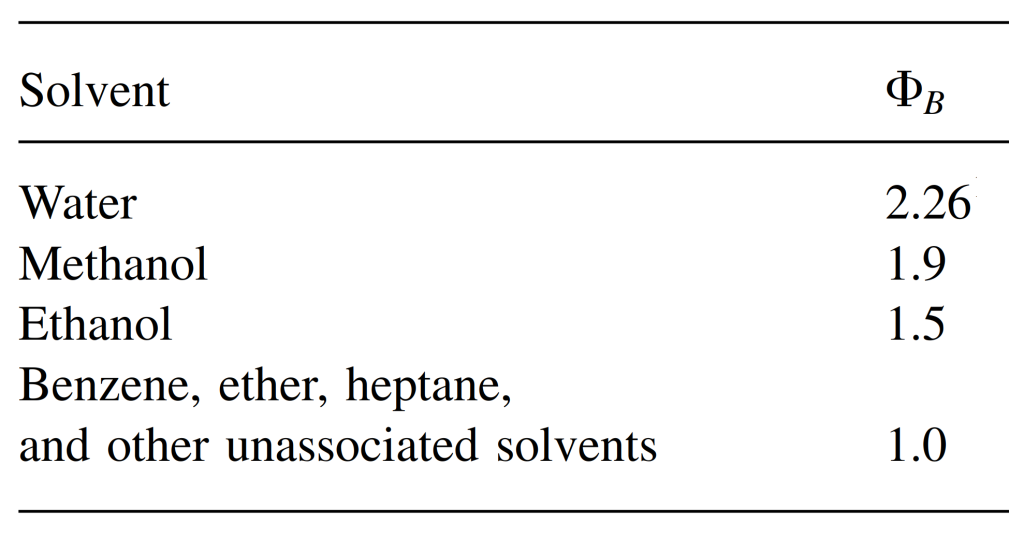
Hayduk-Laudie Correlation
A simpler alternative, mainly used for non-electrolytes in water, is the Hayduk-Laudie correlation (Equation 24-53). It’s similar to the Wilke-Chang correlation and is useful for estimating the diffusivity of solutes in very dilute solutions.
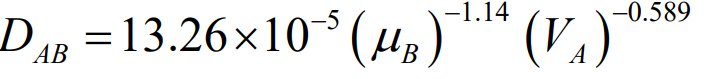
Note that these correlations are accurate for solutions that are not too concentrated, as the liquid phase diffusivities are more sensitive to concentration changes compared to gases.
Taylor and Duss Technique
For non-dilute solutions, Taylor and Duss (2019) suggest a general approach, applicable to both gas and liquid mixtures. This involves combining all species other than the key solute (A) into a single group (B) and calculating their combined properties using specific equations. This method is also adaptable for the liquid phase in both Wilke-Chang and Hayduk-Laudie correlations.
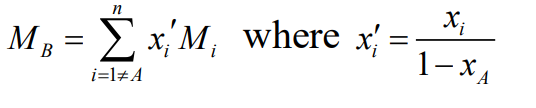
Remember, when applying these correlations to mixtures, use a weighted average for the properties of species B, excluding the key solute (A). A similar weighted result is used for the “molecular volume” of B, if needed in the correlation.
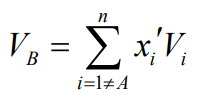
In conclusion, mastering the art of estimating binary diffusivity in liquids is a crucial skill in the field of chemical engineering. By adeptly applying the Wilke-Chang and Hayduk-Laudie correlations, and employing the advanced techniques suggested by Taylor and Duss for more complex mixtures, you can navigate through the intricacies of diffusion in liquid solutions with confidence. Remember, the key lies in understanding the principles, adapting to the specifics of your solution, and applying the knowledge with precision. So, dive into your calculations with enthusiasm, and let the dynamic world of diffusivity unfold before you! Keep exploring, keep learning, and most importantly, keep innovating! 🔍📊🌊💡🚀
References
- R. Taylor and M. Duss, Industrial and Engineering Chemistry Research, 58, 16877-16893 (2019).
- J. Welty, C.E. Wicks, G.L. Rorrer, and R.E. Wilson, Fundamentals of Momentum, Heat, and Mass Transfer, 5th edition, Wiley, Hoboken, NJ (2007).
- R. Shankar Subramanian, Lecture Notes on the Estimation of Binary Diffusivities (2020)
- Poling, Bruce E. The properties of gases and liquids (2004).

